SolutionShow Solution On a graph paper, draw a horizontal line X'OX and a vertical line YOY' as the xaxis and yaxis, respectively ∴ `y = (2x)/2` (i) Putting x = 2, we get y = 0 Putting x = 0, we get y = 1 Putting x = 2, we get y = 2 Thus, we have the following table for the equation x 2y 2 x = 7, y = 1 First, choose one of the 2 equations x 6y = 13 eq 1 2x 6y = 8 eq 2 For this answer i chose x 6y = 13 the first thing i'm going to do is to isolate the x, transfer 6y to the right side of the equal sign Then it becomes x = 13 6y eq 1 *noticed that 6y becomes negative when transferredSolve by Graphing xy=2 , xy=8, Subtract from both sides of the equation Multiply each term in by Tap for more steps Multiply each term in by Multiply Tap for more steps Multiply by Multiply by Simplify each term Tap for more steps Multiply by Multiply Tap for more steps
16 X Y 2 X Y 1 8 X Y 12 X Y 7 Find The Value Of X And Y Quora
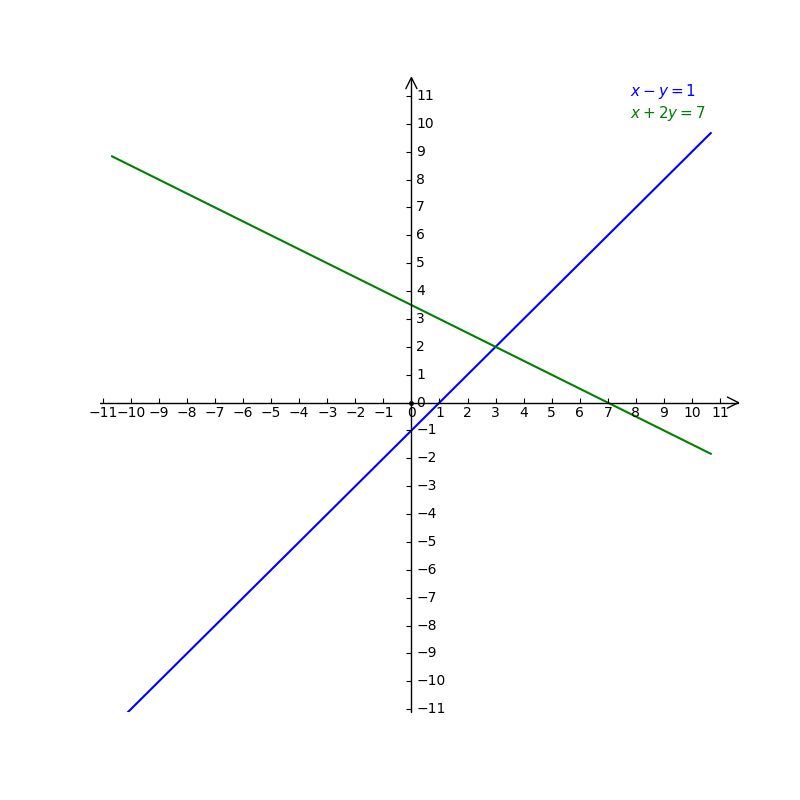
X+y=8 x-y=2 graphical method
X+y=8 x-y=2 graphical method- Transcript Example 15 Solve the following system of inequalities graphically x 2y ≤ 8 , 2x y ≤ 8 , x ≥ 0 , y ≥ 0 First we solve x 2y ≤ 8 Lets first draw graph of x 2y = 8 Putting x = 0 in (1) 0 2y = 8 2y = 8 y = 8/2 y = 4 Putting y = 0 in (1) x 2(0) = 8 x 0 = 8 x = 8 Points to be plotted are (0,4) , (8,0) Drawing graph Checking for (0,0) Putting x = 0, y = 0 x 2y ≤ 3 Graphical Solution of a System of Linear Equations A `2 ×2` system of equations is a set of 2 equations in 2 unknowns which must be solved simultaneously (together) so that the solutions are true in both equations We can solve such a system of equations graphicallyThat is, we draw the graph of the 2 lines and see where the lines intersect




Graphing A Linear Equation 5x 2y Video Khan Academy
Draw the first three constraints, which are lines You'll get a shape with straight sides (two of which are the x and yaxes) and a certain number of corners, one of which is (0,0) I didn't actually graph those particular lines, so I don't knowX2 0 Goal produce a pair of x1 and x2 that (i) satis es all constraints and (ii) has the greatest objectivefunction valueType 1 Two Linear Graphs It is possible to solve linear simultaneous equations with graphs by finding where they intersect Example By plotting their graphs, for values of x between 1 and 4, on the same axes, find the solution to the two simultaneous equations below \begin{aligned}y&=2x5 \\ y&=x4\end{aligned}
3x y = 8 (i) 6x 2y = 16 (ii) Putting x = 0 in equation (i) we get `=> 3 xx 0 y = 8` `=> y = 8` x = 0, y = 8 Putting y = 0 in equations (i) we get `=> 3x 0 = 8` => x = 8/3 x = 8/3, y = 0 see below Graphically the roots are where the graph crosses the xaxis that is when y=0 graph{x^28x16 374, 1404, 256, 633} As can be seen from the graph it touches the xaxis at one point only x=4 Algebraically we could use factorising, completing the square or the formula look for factorising first x^28x=16=0 (x4)^2=(x4)(x4)=0 x4=0=>x=4 theVit C 2x y !
Now plot y = x/2 3 (as a solid line because y≤ includes equal to) 3 Shade the area below (because y is less than or equal to) Example y/2 2 > x 1 We will need to rearrange this one so "y" is on its own on the left Start with y/2 2 > x Subtract 2 from both sides y/2 > x − 2Solve the following pair of linear equations using Graphical method x y = 8;Observe that, given any values for x3 and x4, the values of x1 and x2 are determined uniquely by the equalities In fact, setting x 3 = x 4 = 0 immediately gives a feasible solution with x 1 = 6 and x 2



Solve The Simultaneous Linear Equations X Y 1 And Gauthmath




Solve Following Simultaneous Equation By Using Graphical Method X Y 8 X Y 2 Brainly In
#SahajAdhyayan #सहजअध्ययन #graphically Class 10 (इयत्ता 10वी ) Practice set 12 (सराव संच 12) Graphical Method x y = 0 ;SOLUTION xy=8;xy=2 simeltenious equations using graphical method Algebra > Graphs > SOLUTION xy=8;xy=2 simeltenious equations using graphical method Log On Algebra Graphs, graphing equations and inequalities Section8 iron 2x 2y !
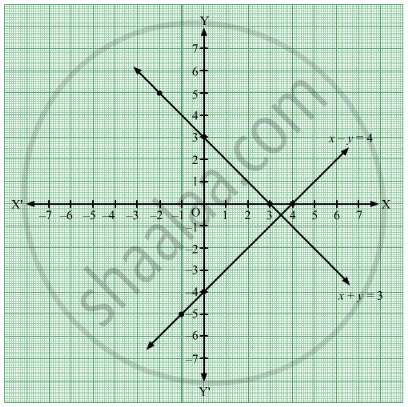



Complete The Following Table To Draw Graph Of The Equations I X Y 3 Ii X Y 4 Algebra Shaalaa Com



Solution X Y 8 X Y 2 Simeltenious Equations Using Graphical Method
Use graph paper for this question Take 2 cm = 2 units on xaxis and 2 cm = 1 unit on yaxis Solve graphically the following equations 3x 5y = 12;1 Using graphical method, solve the following linear programming problem Minimize T x y = 3 Subject to x 2y ≥ 4 x 3y ≥ 6 x ≥ 0 y ≥ 0 5 marks 2 A company needs to purchase a number of printing machines of which there are two types X and Y Type X costs shillings 000 and requires two operators and occupies 8 sq mtrs of floor spaceSolve the following pair of linear equations using Graphical method x y = 8;




Q3e 3x Y 10 X Y 2 Solve The Following Simultaneous Equation Graphically




Graphical Method Tilted Smith Charts In X Y Plane Download Scientific Diagram
0 Cost = C = 4x3y The 3 blue lines are 48 = 4x3y 36 = 4x3y 24 = 4x3y The cost will be minimized if the strategy followed is the one corresponding to this corner point vit c x = #oz of A y = #oz of BThe simultanous equation calculator helps you find the value of unknown varriables of a system of linear, quadratic, or nonlinear equations for 2, 3,4 or 5 unknowns A system of 3 linear equations with 3 unknowns x,y,z is a classic example This solve linear equation solver 3 unknowns helps you solve such systems systematicallyTo find the yintercept (s), substitute in for and solve for Solve the equation Tap for more steps Remove parentheses Add and yintercept (s) in point form yintercept (s) yintercept (s) Create a table of the and values Graph the line using the slope and the yintercept, or the points




Equation Xy Yx Wikipedia
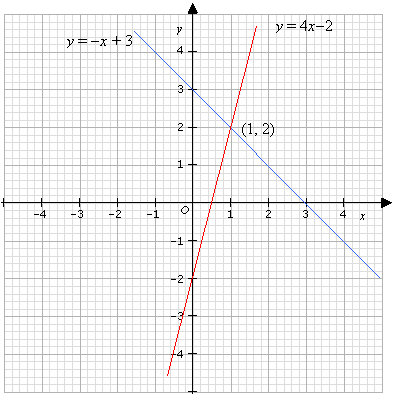



Systems Of Equations Graphical Method Video Lessons Examples Solutions
X y = 2 Then (x, y) is equal to 10th Maths Pair of Linear Equations in Two VariablesSolve for X and Y the equations are 2 X 3 Y is equal to 9 and 4 x 6 Y is equal to 15Thus, the line BC is the graph of 2x 3y = 2 Graph of x 2y = 8 x – 2y = 8 ⇒ 2y = (x – 8) ⇒ `y=(x8)/2` (ii) Putting x = 2, we get y = 3 Putting x = 4, we get y = 2 Putting x = 0, we get y = 4 Thus, we have the following table for the equation x – 2y = 8




X Y 8 X Y 2 Solution Of This Sum In Graphical Method Brainly In



Solve The Following Simultaneous Equations Graphically I 3x Y 2 0 2x Y 8 Ii 3x Y 10 X Y 2 Sarthaks Econnect Largest Online Education Community



0 件のコメント:
コメントを投稿